Tapering and implied volume equations for Pinus leiophylla Schiede ex Schltdl. & Cham. in state of Michoacán
DOI:
https://doi.org/10.29298/rmcf.v16i89.1508Keywords:
Clutter, mercado diferenciado, perfil fustal, efectos mixtos, Schumacher-Hall, aprovechamiento forestalAbstract
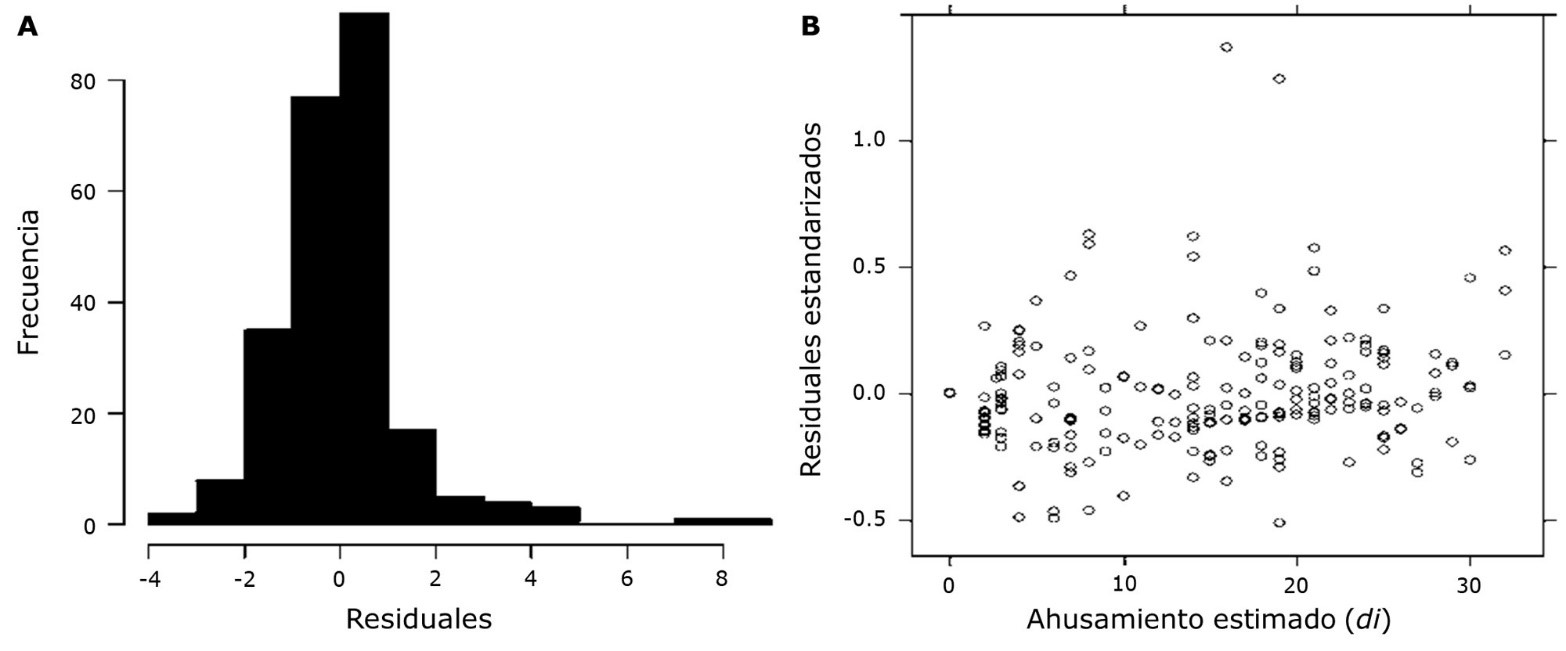
The description of the taper (di) and the precise estimation of the stem volume (sV) are fundamental in the estimation of the timber stock and the distribution of products. The objective of the study was to fit a taper function and define the implicit sV model for Pinus leiophylla trees in the indigenous community of Patambán, Tangancícuaro, state of Michoacán, Mexico. Eight taper functions were fitted using data from 36 dominant trees from forest plantations (245 normal diameter [d], diameters [di] and heights [Ai] at different stem sections). Mixed effects models (MEM) and the maximum likelihood technique were utilized to correct for heteroscedasticity and self-correlation with the expression varExp: and a moving average (ARMA) structure of order (p, q), respectively. Statistically, Clutter’s taper expression: was more accurate as it included the random effect ( ) additively in the total height parameter (Th). The explanation of sampling variability was 97.8 %, with an overall estimation error of 1.406 cm and an individual bias of 0.0138 cm. The implicit sV model was Schumacher-Hall: (d and Th in m). The proposed expressions can be reliably included in the description of inventory data or yield estimates for forest crops.
Downloads
References
Balzarini, M. (2002). Applications of mixed models in plant breeding. In M. S. Kang (Ed.), Quantitative genetics, genomics and plant breeding (pp. 353-363). CABI Publishing. https://www.researchgate.net/publication/286931755_Applications_of_Mixed_Models_in_Plant_Breeding DOI: https://doi.org/10.1079/9780851996011.0353
Bandera F., E., y Pérez P., L. (2018). Los modelos lineales generalizados mixtos. Su aplicación en el mejoramiento de plantas. Cultivos Tropicales, 39(1), 127-133. https://www.redalyc.org/articulo.oa?id=193260614019
Baty, F., Ritz, C., Charles, S., Brutsche, M., Flandrois, J.-P., & Delignette-Muller, M.-L. (2015). A toolbox for nonlinear regression in R: The rackage nlstools. Journal of Statistical Software, 66(5), 1-21. https://www.jstatsoft.org/article/view/v066i05 DOI: https://doi.org/10.18637/jss.v066.i05
Bronisz, K., & Mehtätalo, L. (2020). Mixed-effects generalized height–diameter model for young silver birch stands on post-agricultural lands. Forest Ecology and Management, 460, Article 117901. https://doi.org/10.1016/j.foreco.2020.117901 DOI: https://doi.org/10.1016/j.foreco.2020.117901
Çakir, C. Y., & Kahriman, A. (2018). Modeling stem profile of Caucasian fir and Oriental spruce mixed stands in Turkey using nonlinear mixed-effects models. Applied Ecology and Environmental Research, 16(5), 6815-6833. https://aloki.hu/pdf/1605_68156833.pdf
Correa M., J. C., y Salazar U., J. C. (2016). Introducción a los modelos mixtos. Universidad Nacional de Colombia. https://medellin.unal.edu.co/todos-los-libros/images/gratuitos/pdf/Introduccion-a-los-modelos-Mixtos_Mayo_2017.pdf
Cruz-Cobos, F., De los Santos-Posadas, H. M., y Valdez-Lazalde, J. R. (2008). Sistema compatible de ahusamiento-volumen para Pinus cooperi Blanco en Durango, México. Agrociencia, 42(4), 473-485. https://agrociencia-colpos.org/index.php/agrociencia/article/view/646
Cruz-Cobos, F., Quiñonez-Barraza, G., Hernández-Merino, V., Corral-Rivas, S., y Nava-Nava, A. (2023). Sistemas compatibles de ahusamiento y volumen comercial para dos especies de Pinus en Durango, México. Revista Mexicana de Ciencias Forestales, 14(77), 76-102. https://doi.org/10.29298/rmcf.v14i77.1383 DOI: https://doi.org/10.29298/rmcf.v14i77.1383
Flores M., E. A., Rodríguez A., A. C., Aguirre C., O. A., Alanís R., E., y Quiñonez B., G. (2021). Sistema compatible de ahusamiento-volumen para Pinus pseudostrobus Lindl. en el ejido Corona del Rosal, Nuevo León, México. Madera y Bosques, 27(2), Artículo e2722130. https://doi.org/10.21829/myb.2021.2722130 DOI: https://doi.org/10.21829/myb.2021.2722130
Fu, L., Wang, M., Lei, Y., & Tang, S. (2014). Parameter estimation of two-level nonlinear mixed effects models using first order conditional linearization and the EM algorithm. Computational Statistics & Data Analysis, 69, 173-183. https://doi.org/10.1016/j.csda.2013.05.026 DOI: https://doi.org/10.1016/j.csda.2013.05.026
García-Espinoza, G. G., Aguirre-Calderón, O. A., Vargas-Larreta, B., Martínez-Ángel, L., García-Magaña, J. J., y Hernández-Ramos, J. (2019). Sistema compatible de ahusamiento y volumen comercial de Pinus pseudostrobus Lindl. en Nuevo San Juan Parangaricutiro, Michoacán, México. Agrociencia, 53(1), 115-131. https://agrociencia-colpos.org/index.php/agrociencia/article/view/1755
Hernández P., D., de los Santos P., H. M., Ángeles P., G., Valdez L., J. R., y Volke H., V. H. (2013). Funciones de ahusamiento y volumen comercial para Pinus patula Schltdl. et Cham. en Zacualtipán, Hidalgo. Revista Mexicana de Ciencias Forestales, 4(16), 34-45. https://doi.org/10.29298/rmcf.v4i16.439 DOI: https://doi.org/10.29298/rmcf.v4i16.439
Hernández-Ramos, J., De los Santos-Posadas, H. M., Valdéz-Lazalde, J. R., Tamarit-Urias, J. C., Ángeles-Pérez, G., Hernández-Ramos, A., y Peduzzi, A. (2018). Funciones de ahusamiento para clones de Eucalyptus urophylla establecidos en plantaciones comerciales en Huimanguillo, Tabasco, México. Agrociencia, 52(7), 1013-1029. https://agrociencia-colpos.org/index.php/agrociencia/article/view/1719/1719
Hernández-Ramos, J., Hernández-Ramos, A., García-Magaña, J. de J., García-Cuevas, X., García-Espinoza, G. G., Muñoz-Flores, H. J., y Olvera-Delgadillo, E. H. (2017). Sistema compatible de ahusamiento-volumen comercial para plantaciones de Pinus greggii Engelm. en Hidalgo, México. Revista Mexicana de Ciencias Forestales, 8(39), 59-70. https://doi.org/10.29298/rmcf.v8i39.43 DOI: https://doi.org/10.29298/rmcf.v8i39.43
Infante G., S., y Zárate de L., G. P. (2012). Métodos estadísticos: un enfoque interdisciplinario (3ra Edición). Editorial del Colegio de Postgraduados. https://archive.org/details/libro-metodos-estadisticos-infante-y-zarate-2/page/n1/mode/2up
Instituto Nacional de Estadística y Geografía. (2010). Compendio de información geográfica municipal de los Estados Unidos Mexicanos. Tangancícuaro, Michoacán de Ocampo, clave geoestadística 16085. Instituto Nacional de Estadística y Geografía. https://www.inegi.org.mx/contenidos/app/mexicocifras/datos_geograficos/16/16085.pdf
Martínez-González, M. A., Sánchez-Villegas, A., Toledo A., E., y Faulin F., J. (Edits.). (2014). Bioestadística amigable (3ra Edición). Elsevier España, S. L. https://www.unav.edu/documents/16089811/16155256/indice-bioestadistica-amigable-3-edicion.pdf
McTague, J. P., & Weiskittel, A. (2021). Evolution, history, and use of stem taper equations: a review of their development, application, and implementation. Canadian Journal of Forest Research, 51(2), 210-235. https://doi.org/10.1139/cjfr-2020-0326 DOI: https://doi.org/10.1139/cjfr-2020-0326
Monárrez-González, J. C., Márquez-Linares, M. A., López H., J. A., Pérez V., G., Quiñonez B., G., y García C., X. (2024). Ecuaciones de volumen fustal-total y ahusamiento para especies maderables del ecosistema templado en Puebla, México. Revista Mexicana de Ciencias Forestales, 15(84), 4-28. https://doi.org/10.29298/rmcf.v15i84.1440 DOI: https://doi.org/10.29298/rmcf.v15i84.1440
Niño L., G. S., Ramos M., P. A., Barrios, A., y López A., A. M. (2018). Modelos compatibles de ahusamiento-volumen para árboles de Gmelina arborea Roxb. en el Alto Magdalena, Colombia. Colombia Forestal, 21(2), 174-187. https://doi.org/10.14483/2256201X.12249 DOI: https://doi.org/10.14483/2256201X.12249
Pinheiro, J. C., & Bates, D. M. (2000). Mixed-effects models in S and S-PLUS. Springer. https://doi.org/10.1007/b98882 DOI: https://doi.org/10.1007/978-1-4419-0318-1
Pinheiro, J. C., Bates, D., DebRoy, S., Sarkar, D., Heisterkamp, S., Van Willigen, B., & Ranke, J. (2025). Linear and Nonlinear Mixed Effects Models. Package ‘nlme’: Version 3.1-168. Comprehensive R Archive Network. https://cran.r-project.org/web/packages/nlme/nlme.pdf
Pompa G., M., Corral R., J. J., Díaz V., M. A., y Martínez S., M. (2009). Función de ahusamiento y volumen compatible para Pinus arizonica Engelm. en el suroeste de Chihuahua. Revista Ciencia Forestal en México, 34(105), 119-136. https://cienciasforestales.inifap.gob.mx/index.php/forestales/article/view/695
R Core Team. (2024). RStudio Builds 2024.04.2 (version Build 764) [Software]. R Foundation for Statistical Computing. https://dailies.rstudio.com/version/2024.04.2+764/
Rachid C., C., Mason E., G., Wollons, R., & Resquin, F. (2014). Volume and taper equations for P. taeda (L.) and E. grandis (Hill ex. Maiden). Agrociencia Uruguay, 18(2), 47-60. http://www.ainfo.inia.uy/digital/bitstream/item/13335/1/Rachid-Agrociencia-2014.pdf DOI: https://doi.org/10.31285/AGRO.18.464
Ramírez-Martínez, A., Santiago-García, W., Quiñonez-Barraza, G., Ruiz-Aquino, F., y Antúnez, P. (2018). Modelación del perfil fustal y volumen total para Pinus ayacahuite Ehren. Madera y Bosques, 24(2), Artículo e2421496. https://doi.org/10.21829/myb.2018.2421496 DOI: https://doi.org/10.21829/myb.2018.2421496
Saygili, B., & Kahriman, A. (2023). Modeling compatible taper and stem volume of pure Scots pine stands in Northeastern Turkey. iForest, 16(1), 38-46. https://doi.org/10.3832/ifor4099-015 DOI: https://doi.org/10.3832/ifor4099-015
Şenyurt, M., Ercanlı, İ., & Bolat, F. (2017). Taper equations based on nonlinear mixed effect modeling approach for Pinus nigra in Çankırı forests. Bosque, 38(3), 545-554. http://dx.doi.org/10.4067/S0717-92002017000300012 DOI: https://doi.org/10.4067/S0717-92002017000300012
Shin, J.-H., Han, H., Ko, C.-U., Kang, J.-T., & Kim, Y.-H. (2022). Applying nonlinear mixed-effects models to taper equations: a case study of Pinus densiflora in Gangwon province, Republic of Korea. Journal of Korean Society of Forest Science, 111(1), 136-149. https://doi.org/10.14578/jkfs.2022.111.1.136
Tamarit U., J. C., De los Santos P., H. M., Aldrete, A., Valdez L., J. R., Ramírez M., H., y Guerra De la C., V. (2014). Sistema de cubicación para árboles individuales de Tectona grandis L. f. mediante funciones compatibles de ahusamiento-volumen. Revista Mexicana de Ciencias Forestales, 5(21), 58-77. https://doi.org/10.29298/rmcf.v5i21.358 DOI: https://doi.org/10.29298/rmcf.v5i21.358
Torres A., D., de los Santos P., H. M., Velázquez M., A., y Tamarit U., J. C. (2020). Ahusamiento y volumen comercial de tres procedencias de pinos tropicales en plantaciones forestales de Veracruz, México. Madera y Bosques, 26(3), Artículo e2631890. https://myb.ojs.inecol.mx/index.php/myb/article/download/1890/e2631890/12514 DOI: https://doi.org/10.21829/myb.2020.2631890
Yang, Y., & Huang, S. (2013). On the statistical and biological behaviors of nonlinear mixed forest models. European Journal of Forest Research, 132, 727-736. https://doi.org/10.1007/s10342-013-0705-2 DOI: https://doi.org/10.1007/s10342-013-0705-2
Yang, Y., Huang, S., Trincado, G., & Meng, S. X. (2009). Nonlinear mixed-effects modeling of variable-exponent taper equations for lodgepole pine in Alberta, Canada. European Journal of Forest Research, 128, 415-429. https://doi.org/10.1007/s10342-009-0286-2 DOI: https://doi.org/10.1007/s10342-009-0286-2
Zhang, S., Sun, J., Duan, A., & Zhang, J. (2021). Variable-exponent taper equation based on multilevel nonlinear mixed effect for Chinese Fir in China. Forests, 12(2), 126. https://doi.org/10.3390/f12020126 DOI: https://doi.org/10.3390/f12020126
Zuur, A. F., Ieno, E. N., Walker, N., Saveliev, A. A., & Smith, G. M. (2009). Mixed effects models and extensions in ecology with R. Springer. https://link.springer.com/book/10.1007/978-0-387-87458-6 DOI: https://doi.org/10.1007/978-0-387-87458-6
Published
How to Cite
Issue
Section
License
Copyright (c) 2025 Revista Mexicana de Ciencias Forestales

This work is licensed under a Creative Commons Attribution-NonCommercial 4.0 International License.
The authors who publish in Revista Mexicana de Ciencias Forestales accept the following conditions:
In accordance with copyright laws, Revista Mexicana de Ciencias Forestales recognizes and respects the authors’ moral right and ownership of property rights which will be transferred to the journal for dissemination in open access.
All the texts published by Revista Mexicana de Ciencias Forestales –with no exception– are distributed under a Creative Commons License Attribution-NonCommercial 4.0 International (CC BY-NC 4.0), which allows third parties to use the publication as long as the work’s authorship and its first publication in this journal are mentioned
The author(s) can enter into independent and additional contractual agreements for the nonexclusive distribution of the version of the article published in Revista Mexicana de Ciencias Forestales (for example, include it into an institutional repository or publish it in a book) as long as it is clearly and explicitly indicated that the work was published for the first time in Revista Mexicana de Ciencias Forestales.
For all the above, the authors shall send the form of Letter-transfer of Property Rights for the first publication duly filled in and signed by the author(s). This form must be sent as a PDF file to: ciencia.forestal2@inifap.gob.mx
This work is licensed under a Creative Commons Attribution-Noncommercial 4.0 International license.





